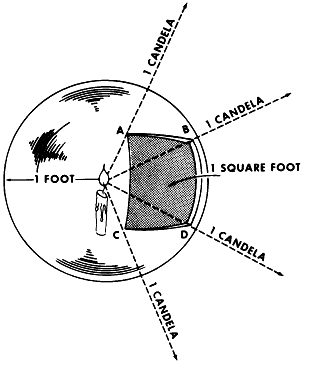
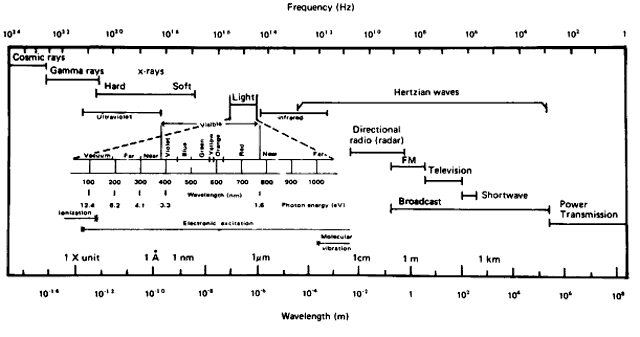
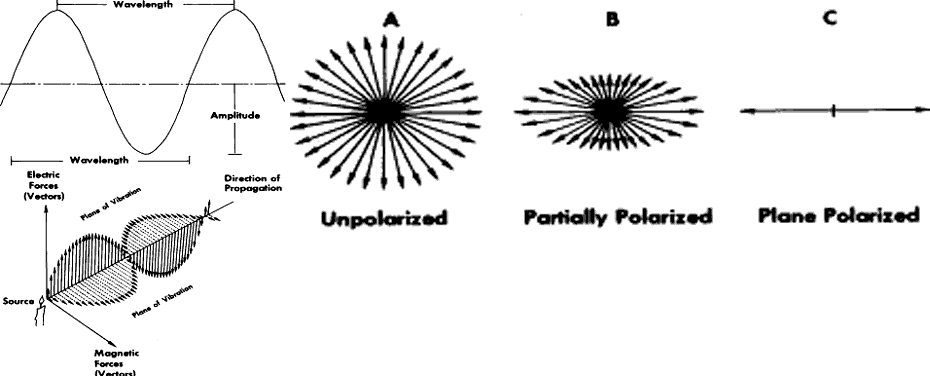
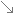A basic characteristic of all electromagnetic energy, including light, is that it is produced by electrons that have been excited by an external force and have just reverted to a more stable position, thereby releasing energy. Such electromagnetic energy travels in a straight line (rectilinear propagation) from one point to another as a series of waves alternating perpendicular to one another and transverse to the direction of propagation (Fig. 2). Light and all other electromagnetic radiation travel at the same speed, which is approximately 3 × 1010 cm/sec in a vacuum. The speed of propagation is only slightly slower in clear air, and the decrease in velocity is inconsequential for physiologic purposes. In denser transparent media, such as the cornea, water, or optical glass, the velocity is much less, and it is this slowing of light rays by the denser media that is described by Snell's law or the refraction law. The ability of glass, plastic, and other transparent material to slow the progress of a beam of light makes possible the construction of lens systems to bring rays of light to a focus or diverge them to correct optical abnormalities of the human eye. The same principles apply to the anterior segment of the eye in its ability to take parallel or diverging rays of light and focus them on the retina to produce a sharp image rather than only an unformed sensation of lightness or darkness. The refractive indices of some common transparent materials are shown in Table 1.
TABLE 1. Refractive Indices of the Eye and Various Common Substances
| Substance | Refractive Index |
| Cornea | 1.376 |
| Aqueous humor | 1.336 |
| Lens | 1.410 |
| Vitreous humor | 1.336 |
| Ice at 0°C | 1.312 |
| Distilled H2 O at 20°C | 1.33 |
| Polymethyl methacrylate | 1.491 |
| Light crown glass | 1.515 |
| Flint glass | 1.570–1.751 |
| Diamond | 2.417 |
Visible light has three important characteristics, one of which is wavelength. This is the distance between any two similar given points on a single wave of light. It is usually recorded as the distance from one crest to the next adjoining crest (see Fig. 2). Wavelength is abbreviated lambda (λ), and of all the physical dimensions it is the most important in determining the color of light. Another important characteristic is the speed of light, which was discussed earlier. The third is frequency, which is usually abbreviated V and is the number of complete cycles moving past a specific point over a given period of time. Velocity, wavelength, and frequency are related in the following manner (equation 1):
Velocity (V) = wavelength (λ) × frequency (ν)
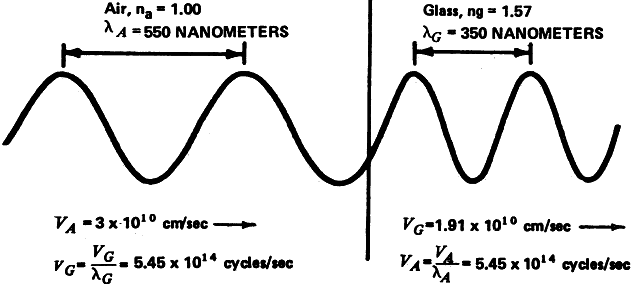
Frequency is a constant, and consequently wavelength and velocity are reciprocally related. If velocity decreases as a ray of light passes through crown glass, then the wavelength must shorten so that the frequency will remain constant (Fig. 3).
The speed of light can be measured in a variety of ways. One simple and direct method of such a measurement is the use of mirrors rotating at a (known speed and separated by a known distance. Wavelength can be measured by an interferometer. This somewhat cumbersome and delicate laboratory instrument uses an interference grating and will measure exactly the wavelength of any given test light. If the wavelength and velocity are known, the frequency is easily calculated from equation 1.
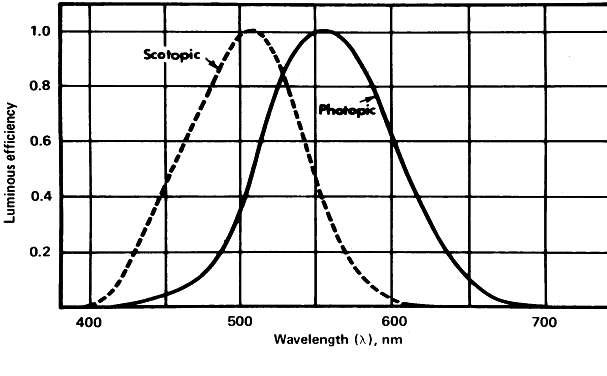
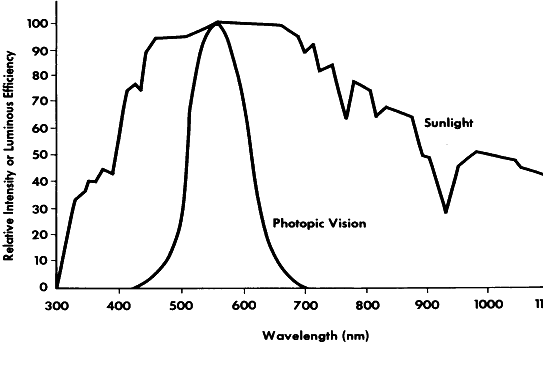
The exact nature of light is still unknown, although a number of theories that have been deduced from the physical behavior of light are useful for descriptive purposes. The foremost theories are those of Newton and Huygens. Newton in 1672 held that light is a particle or corpuscle that travels in a straight line from its source to the eye or from its source to an object, where it is then reflected into the eye. Newton's concept of light is still quite useful today when an attempt is made to describe the physical effects of light that result in transformation of the energy contained in the light ray. An example of this energy transformation is light falling on photographic film or the photochemical effect of light on the retinal pigments or on the basal cells of the skin. The toxic effects of light and its carcinogenic properties are also best explained in terms of particles impacting on a molecule and producing a chemical change therein. Such energy transformation can be considered in terms of light as a nonmaterial particle of minimal energy, a single unit of which is termed a photon or quantum. These terms were coined by Planck, who believed that light energy was released and absorbed in discrete quanta or photons. The retinal photoreceptors do seem to behave as true quantum detectors rather than as simple energy detectors, in that a single quantum of light absorbed by one molecule of retinal pigment located in a photoreceptor outer segment containing some 2 × 108 other unaffected molecules is a sufficient stimulus to activate the receptor.2 The energy for a given quantum of light is least for the longest wavelengths and greatest for the shortest (see Fig. 1). Therefore, the energy contained in a given number of quanta is directly proportional to their frequency and inversely proportional to their wavelength. The energy (E) so contained can be calculated by the following formula (equation 2):
E = h·ν
where h is Planck's constant 6.6256 × 10-27 joule-seconds, and ν is the frequency in hertz. The amount of available energy from the short violet end of the spectrum is about twice that from the longer red end (see Fig. 1). This is an important consideration when determining the heat-generating properties of light. When considering stimulation of the retinal photoreceptors, the total energy present in any given quantum of light is unimportant. The critical factor is the wavelength of the incident light and the ability of the photopigment to absorb it. If a specific wavelength of light can be absorbed by photopigment, the resultant reactions will be of the same magnitude regardless of whether the wavelength is long or short. Generally, Newton's concept (with Planck's modification) of light as a particle or corpuscle is most useful in describing the events that occur in photochemical reactions, photoelectric cell function, and other light-induced chemical or physical reactions.
The wave theory suggested by Huygens in 1678 states that light is generated by molecular vibrations in a luminous material and that these vibrations are transmitted through a transparent medium as waves whose movements are perpendicular to the direction of propagation. Maxwell in 1865 proposed that such wave motion is electromagnetic in nature and that light is similar in nature to all other electromagnetic energy forms that can be transmitted through a vacuum.3 The wave theory is most useful in describing the behavior of light passing through gratings or other small openings and thereby generating interference phenomena. It is also useful in describing the refraction of light by media of varying optical densities, including optical lenses, the cornea, and the lens of the eye. As noted previously, Snell was the first to describe the behavior of a ray of light passing from a medium of one optical density into or through a medium of dissimilar optical density. Snell's description of the behavior of light under these conditions is known as Snell's law or the refraction law. It states that the ratio of the sine of the angle of incidence to the sine of the angle of refraction of a ray of light is equal to the ratio of the velocity in the first medium to the velocity in the second medium. The refractive index of any given optical medium is the ratio of the speed of light in a vacuum to the speed of light in the material in question. Using the refractive index in Snell's equation rather than the velocity greatly simplifies the resulting calculation (equation 3):
n1 sine1 = n2 sine2
Here, n1 is the index of refraction of the first medium, sine1 is the sine of the angle of incidence, n2 is the index of refraction of the second optical medium, and sine2 is the sine of the angle of the refracted ray. Snell's law is one of the fundamental principles of geometric and physiologic optics.
 )
) K(λ)Qeλdλ
K(λ)Qeλdλ